
Gonçalves, Federal Center of Technological Education of Minas Gerais, Av. Amazonas, Belo Horizonte, Brazil | Ecole Centrale de Lyon, Laboratoire AMPÈRE, Ecully, FranceĬorresponding author: S.T.M. bĪffiliations: Federal Center of Technological Education of Minas Gerais, Av. Guest editors: Paolo Di Barba and Sƚawomir WiakĪuthors: Gonçalves, S.T.M. Letters, 2000, 27: 334–339.Issue title: Selected Papers from ISEF 2015 D., Convolutional PML (CPML): An efficient FDTD implementation of the CFS-PML for arbitrary media, Microw. P., A perfectly matched layer for the absorption of electromagnetic waves, J. H., Application of CPML to Truncate the Open Boundaries of 3D Rectangular Waveguides, High Power Laser and Particle Beams, 2005, in print.īérenger, J.
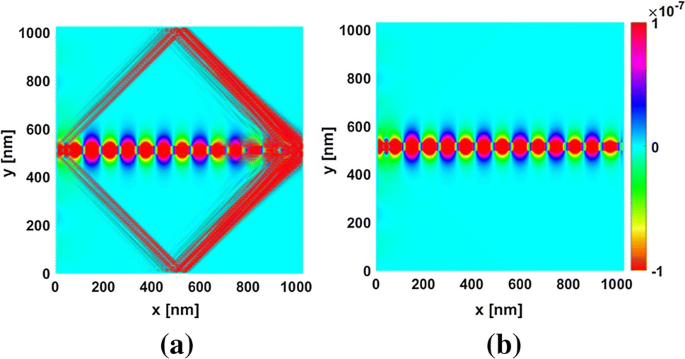
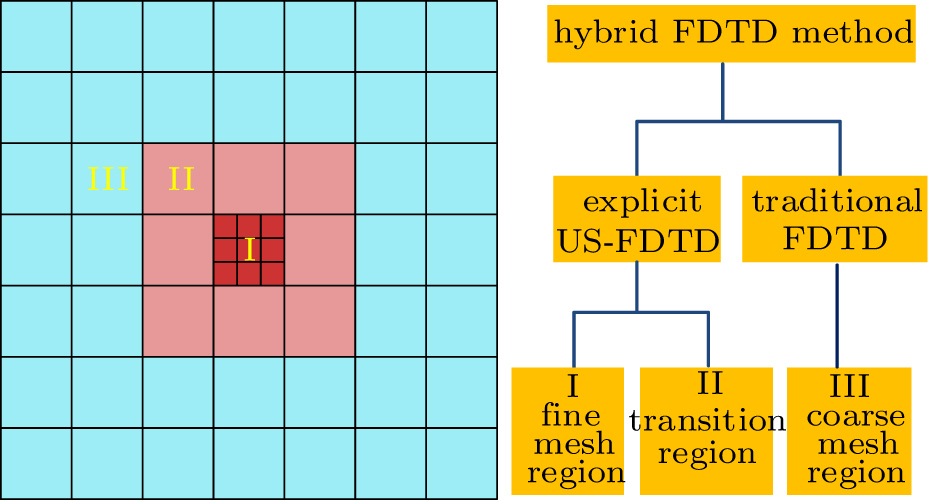
P., User’s Manual for Code Karat, Moscow: Aug. Mur, G., Absorbing boundary conditions for the finite-difference approximation of the time-domain electromagnetic-field equations, IEEE Trans. Wang Jianguo, Liu Guozhi, Zhang Dianhui, PIC method for numerical simulation of HPM sources, Test and Research, 2003, 26(1): 97–104. J., Schamiloglu, E., High Power Microwave Sources and Technology, New York: IEEE Press, 2001. The CPML is especially suitable for truncating the open boundaries of the dispersive waveguide devices in the simulation of HPM sources.īarker, R. The results show that the property of CPML is much better than that of the Mur-type absorbing boundary condition when they are used to truncate the open boundaries of waveguides. the CPML method is introduced in the 2.5-dimensional electromagnetic PIC software, and the relativistic backward wave oscillator is simulated by using this method. Numerical results show that the maximum relative errors are all less than −90 dB. The performance of CPML is simulated when it is used to truncate the cylindrical waveguides excited by the sources with different frequencies and modes in the 2.5-dimensional problems. Numerical experiments are conducted to validate the equations and axis boundary conditions. The electromagnetic field’s FDTD equations and the expressions of axis boundary conditions are presented. In order to solve the problem of truncating the open boundaries of cylindrical waveguides used in the simulation of high power microwave (HPM) sources, this paper studies the convolutional PML (CPML) in the cylindrical coordinate system.


 0 kommentar(er)
0 kommentar(er)
